ME-216-F FLUID MECHANICS LAB
L T P Sessional : 25 Marks
- - 2 Practical/Viva : 25 Marks
Total : 50 Marks
Duration of Exam: 3 Hrs.
List of Experiments:
1. To determine the coefficient of impact for vanes.
2. To determine coefficient of discharge of an orifice meter.
3. To determine the coefficient of discharge of Notch (V and Rectangular types ).
4. To determine the friction factor for the pipes.
5. To determine the coefficient of discharge of venturimeter.
6. To determine the coefficient of discharge, contraction & velocity of an orifice.
7. To verify the Bernoulli’s Theorem.
8. To find critical Reynolds number for a pipe flow.
9. To determine the meta-centric height of a floating body.
10. To determine the minor losses due to sudden enlargement, sudden contraction and bends.
11. To show the velocity and pressure variation with radius in a forced vertex flow.
12. To verify the momentum equation.
Note:
1. At least ten experiments are to be performed in the semester.
2. At least eight experiments should be performed from the above list. Remaining two experiments may either be performed from the above list or designed & set by the concerned institute as per the scope of the syllabus.
EXPERIMENT NO:1
Aim: To determine the co efficient of impact for vanes
Apparatus: Collecting tank, Nozzle of given diameter, Vanes of different shape (flat, inclined or curved).
Theory: Momentum equation is based on Newton’s second law of motion which states that the algebraic sum of external forces applied to control volume of fluid in any direction is equal to the rate of change of momentum in that direction. The external forces include the component of the weight of the fluid & of the forces exerted externally upon the boundary surface of the control volume. If a vertical water jet moving with velocity is made to strike a target, which is free to move in the vertical direction then a force will be exerted on the target by the impact of jet, according to momentum equation this force (which is also equal to the force required to bring back the target in its original position) must be equal to the rate of change of momentum of the jet flow in that direction.
Formula Used:
F=ρ Q v(1-cosβ)
F=ρ Q2 (1-cosβ)/A as v=Q/A
Where
F =force (calculated)
ρ = density of water
β=angle of vane
V =velocity of jet
Q =discharge
A =area of nozzle( π/4d2)
(i) for flat vane β=90o
F’ = ρQ2/A
(ii) for hemispherical vane β=180o
for % error =F- F'/ F'x100
F’ = 2 ρQ2/A
F = Force (due to putting of weight)
(iii) for inclined vane
F'=ρ Q v(1-cosβ)
F'=ρ Q2 (1-cosβ)/A
Procedure:
1. Note down the relevant dimension or area of collecting tank, dia of nozzle, and density of water.
2. Install any type of vane i.e. flat, inclined or curved.
3. Note down the position of upper disk, when jet is not running.
4. Note down the reading of height of water in the collecting tank.
5. As the jet strike the vane, position of upper disk is changed, note the reading in the scale to which vane is raised.
6. Put the weight of various values one by one to bring the vane to its initial position.
7. At this position finds out the discharge also.
8. The procedure is repeated for each value of flow rate by reducing the water supply.
9. This procedure can be repeated for different type of vanes and nozzle.
Observations & Calculations:
Dia of nozzle =
Mass density of water ρ =
Area of collecting tank =
Area of nozzle =
Horizontal flat vane
When jet is not running, position of upper disk is at =
SNO | Discharge measurement | Balancing | Theoretical Force F'= ρQ2/A | Error in % = F-F'/F' | ||||
Initial (cm) | Final (cm) | Time (sec) | Discharge (cm3/sec) Q | Mass W (gm) | Force F | |||
Inclined vane
When jet is not running, position of upper disk is at =
Angle of inclination β = 450
| |||||||||||||||||||||||||||||||||||||||||||
Curved hemispherical vane
When jet is not running, position of upper disk is at =
SNO | Discharge measurement | Balancing | Theoretical Force F'= 2ρQ2/A | Error in % = F-F'/F' | ||||
Initial (cm) | Final (cm) | Time (sec) | Discharge (cm3/sec) Q | Mass W (gm) | Force F | |||
Conclusion: Hence the co efficient of impact for vanes is determined.
EXPERIMENT NO:2
Aim: To determine the coefficient of discharge of Orifice meter.
Apparatus: Orifice meter, installed on different pipes, arrangement of varying flow rate, U- tube manometer, collecting tank.
Theory: Orifice meter are depending on Bernoulli’s equation. Orifice meter is a device used for measuring the rate of fluid flowing through a pipe.
Formula Used:
Where
A = Cross section area of inlet
a = Cross section area of outlet
Δh = Head difference in manometer
Q = Discharge
Cd = Coefficient of discharge
g = Acceleration due to gravity
Coefficient of discharge of orifice meter will be less than 1, but smaller than Cd value of venturimeter.
Procedure:
1. Set the manometer pressure to the atmospheric pressure by opening the upper valve.
2. Now start the supply at water controlled by the stop valve.
3. One of the valves of any one of the pipe open and close all other of three.
4. Take the discharge reading for the particular flow.
5. Take the reading for the pressure head on from the u-tube manometer for corresponding reading of discharge.
6. Now take three readings for this pipe and calculate the Cd for that instrument using formula.
7. Now close the valve and open valve of other diameter pipe and take the three reading for this.
8. Similarly take the reading for all other diameter pipe and calculate Cd for each.
Observations & Calculations:
Diameter of Orifice meter =
Area of cross section =
Area of collecting tank =
Discharge | Manometer Reading | Cd= Q √ A2 - a2 Aa√2g∆h | |||||||
Initial reading | Final reading | Difference | Time (sec) | Q | h1 | h2 | h2-h1 | Δh= 13.6(h2-h1) | |
Conclusion: Hence the coefficient of discharge of Orifice meter is __________.
EXPERIMENT NO:3
Aim: To determine the coefficient of discharge of Notch (V, Rectangular and Trapezoidal types).
Apparatus: Arrangement for finding the coefficient of discharge inclusive of supply tank, collecting tank, pointer, scale & different type of notches
Theory: Notches are overflow structure where length of crest along the flow of water is accurately shaped to calculate discharge.
Procedure:
1. The notch under test is positioned at the end of tank with vertical sharp edge on the upstream side.
2. Open the inlet valve and fill water until the crest of notch.
3. Note down the height of crest level by pointer gauge.
4. Change the inlet supply and note the height of this level in the tank.
5. Note the volume of water collected in collecting tank for a particular time and find out the discharge.
6. Height and discharge readings for different flow rate are noted.
Observations & Calculations:
Breath of tank =
Length of tank =
Height of water to crest level for rectangular notch is =
Height of water to crest level for V notch =
Height of water to crest level for Trapezoidal notch =
Angle of V notch =
Width of Rectangular notch =
Type Of notch | Discharge | Final height reading above width | Head above crest level | Cd | ||||
Initial height Of tank | Final height Of tank | Difference In height | Volume | Q | ||||
Conclusion:
Hence
The coefficient of discharge of V Notch is ______________.
The coefficient of discharge of Rectangular Notch is ______________.
The coefficient of discharge of Trapezoidal Notch is ______________.EXPERIMENT NO:4
Aim: To determine the friction factor for the pipes.(Major Losses).
Apparatus: A flow circuit of G. I. pipes of different diameters, U-tube differential manometer, collecting tank.
Theory:
Friction factor in pipes or Major losses:-
A pipe is a closed conduit through which fluid flows under the pressure. When in the pipe, fluid flows, some of potential energy is lost to overcome hydraulic resistance which is classified as follows:
1. The viscous friction effect associated with fluid flow.
2. The local resistance which result from flow disturbances caused by
a. Sudden expansion and contraction in pipe
b. Obstruction in the form of valves, elbows and other pipe fittings.
c. Curves and bend in the pipe.
d. Entrance and exit losses
The viscous friction loss or major loss in head potential energy due to friction is given by
Procedure:
1. Note down the relevant dimensions as diameter and length of pipe between the pressure tapping, area of collecting tank etc.
2. Pressure tapping of a pipe is kept open while for other pipe is closed.
3. The flow rate was adjusted to its maximum value. By maintaining suitable amount of steady flow in the pipe.
4. The discharge flowing in the circuit is recorded together with the water level in the left and right limbs of manometer tube.
5. The flow rate is reduced in stages by means of flow control valve and the discharge &
reading of manometer are recorded.
6. This procedure is repeated by closing the pressure tapping of this pipe, together with other pipes and for opening of another pipe.
Observations & Calculations:
Diameter of pipe d =
Length of pipe between pressure tapping l =
Area of collecting tank =
SNo | Manometer Reading | Discharge Measurement | 2 5 2 F = π gd / 8lQ hf | |||||
Left limb h1 (cm) | Right limb H2 (cm) | Difference of head in terms of water hf =13.6(h1-h2) | Initial (cm) | Final (cm) | Time (sec) | Discharge Q (cm3/sec) | ||
1. | ||||||||
2. | ||||||||
3. | ||||||||
4. | ||||||||
Conclusion:Hence the friction factor for the pipes is F =_________.
EXPERIMENT NO:5
Aim: To determine the coefficient of discharge of Venturimeter.
Apparatus: Venturimeter, installed on different diameter pipes, arrangement of varying flow rate, U- tube manometer, collecting tube tank.
Theory: Venturimeter are depending on Bernoulli’s equation. Venturimeter is a device used for measuring the rate of fluid flowing through a pipe. The consist of three part in short
1. Converging area part
2. Throat
3. Diverging part
Procedure:
1. Set the manometer pressure to the atmospheric pressure by opening the upper valve.
2. Now start the supply at water controlled by the stop valve.
3. One of the valves of any one of the pipe open and close all other of three.
4. Take the discharge reading for the particular flow.
5. Take the reading for the pressure head on from the u-tube manometer for corresponding reading of discharge.
6. Now take three readings for this pipe and calculate the Cd for that instrument using formula.
7. Now close the valve and open valve of other diameter pipe and take the three reading for this.
8. Similarly take the reading for all other diameter pipe and calculate Cd for each.
Observations & Calculations:
Diameter of Venturimeter =
Area of cross section =
Area of collecting tank =
Discharge | Manometer Reading | Cd= Q √ A2 - a2 Aa√2g∆h | |||||||
Initial reading | Final reading | Difference | Time (sec) | Q | h1 | h2 | h2-h1 | Δh= 13.6(h2-h1) | |
Conclusion: Hence the coefficient of discharge of Venturimeter is __________.
EXPERIMENT NO:6
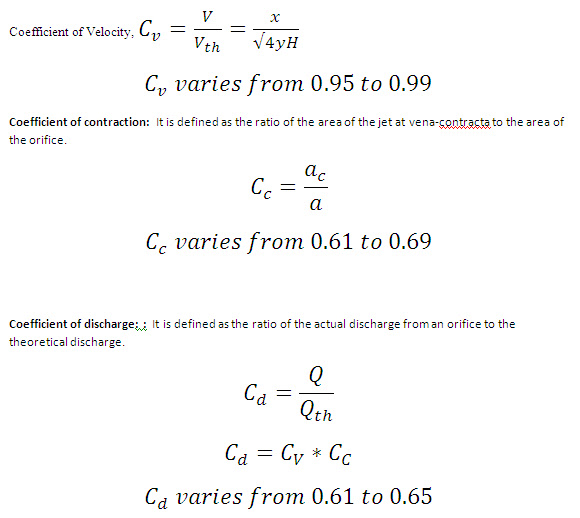
Aim: To determine the coefficient of discharge, contraction & velocity of an Orifice.
Apparatus: Supply tank with overflow arrangement, Orifice plate of different diameter, hook gauge, collecting tank, piezometric tube.
Theory: A mouthpiece is a short length of pipe which is two or three times its diameter in length. If there pipe is filled externally to the orifices, the mouthpiece is called external cylindrical mouthpiece and discharge through orifice increase is a small opening of any cross- section on the side of bottom of the tank, through which the fluid is flowing orifice coefficient of velocity is defined as the ratio of two actual discharge to orifice ratio of the actual velocity of the jet at vena- contracta to the coefficient of theoretical velocity of the jet coefficient of contraction of defined as ratio of the actual velocity of jet at vena- contracta.
Vena- Contracta: The fluid out is in form of jet goes on contracting form orifice up to dispute of about ½ the orifice dia. after the expend this least relation.
Coefficient of velocity: It is a ratio of actual velocity jet at vena-contracta to theoretical velocity.
Procedure:
1. Set the mouthpiece of orifice of which the Cc, Cu, Cd are to be determined.
2. Note the initial height of water in the steady flow tank and the height of datum from the bottom of orifice and mouthpiece. These remains constant for a particular mouthpiece or orifice.
3. By using the stop valve, set a particular flow in tank and tank height of water in tank.
4. Take the reading of discharge on this particular flow.
5. Using hook gauge, find the volume of Xo Y for mouthpiece.
6. Take three readings using hook gauge for one particular orifice.
7. Using the formula get value of Cd, Cu, and Cc for a particular orifice and mouthpiece.
Observations & Calculations:
x' + y' are reading on horizontal/vertical scale
ao | h=µ ao | x' | y' | X= x'-x0 | Y= y'-y0 | CV | Average |
h = Reading on piezometer
a0 = Reading on piezometer at level on centre of mouthpiece
y0 = Reading on vertical scale at exit of orifice
x0 = Reading on horizontal scale at exit of orifice
Sr.No | X | volume | Time | Q = A*V | Cd | Average |
1. | ||||||
2. | ||||||
3. | ||||||
4. |
Conclusion: Hence Cv=________; Cc=________; Cd=________;
EXPERIMENT NO:7
Aim: To verify the Bernoulli’s theorem.
Apparatus: A supply tank of water, a tapered inclined pipe fitted with no. of piezometer tubes point, measuring tank, scale, stop watch.
Theory: Bernoulli’s theorem states that when there is a continues connection between the particle of flowing mass liquid, the total energy of any sector of flow will remain same provided there is no reduction or addition at any point.
Formula Used:
H1 = Z1 + p1/w + V12/2g
H2 = Z2 + p2/w + V22/2g
Procedure:
1. Open the inlet valve slowly and allow the water to flow from the supply tank.
2. Now adjust the flow to get a constant head in the supply tank to make flow in and out flow equal.
3. Under this condition the pressure head will become constant in the piezometer tubes.
4. Note down the quantity of water collected in the measuring tank for a given interval of time.
5. Compute the area of cross-section under the piezometer tube.
6. Compute the area of cross- section under the tube.
7. Change the inlet and outlet supply and note the reading.
8. Take at least three readings as described in the above steps.
Observations & Calculations:
1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | |
Discharge Of piezometer Tube from inlet | |||||||||||
Area of Cross-section Under foot Of each point | |||||||||||
Velocity Of water Under foot Of each point | |||||||||||
V2/2g | |||||||||||
p/ρ | |||||||||||
p/ρ+ V2/2g |
Conclusion: Hence Bernoulli’s theorem is verified.
EXPERIMENT NO:8
Aim: To find critical Reynolds number for a pipe flow.
Apparatus: Flow condition inlet supply, elliptical belt type arrangement for coloured fluid with regulating valve, collecting tank.
Theory: It is defined as the ratio of inertia force of a flowing fluid to the viscous force of the fluid.
Procedure:
1. Fill the supply tank some times before the experiment.
2. The calculated fluid is filled as container.
3. Now set the discharge by using the valve of that particular flow can be obtained.
4. The type of flow of rate is glass tube is made to be known by opening the valve of dye container.
5. Take the reading of discharge for particular flow.
6. Using the formula set the Reynolds no. for that particular flow, aspect the above procedure for all remaining flow.
Observations & Calculations:
Type | Time | Discharge | Q | Re | |||
Initial | Final | Difference | Volume | ||||
EXPERIMENT NO:9
Aim: To determine the Meta-centric height of a floating body.
Apparatus: Take tank 2/3 full of water, floating vessel or pontoon fitted with a pointed pointer moving on a graduated scale, with weights adjusted on a horizontal beam.
Theory: Consider a floating body which is partially immersed in the liquid, when such a body is tilted, the center of buoyancy shifts from its original position ‘B’ to ‘B’ (The point of application of buoyanant force or upward force is known as center of G which may be below or above the center of buoyancy remain same and couple acts on the body. Due to this couple the body remains stable.
At rest both the points G and B also Fb x Wc act through the same vertical line but in opposite direction. For small change (θ) B shifted to B.
The point of intersection M of original vertical line through B and G with the new vertical, line passing through ‘B’ is known as metacentre. The distance between G and M is known as metacentre height which is measure of static stability.
Procedure:
1. Note down the dimensions of the collecting tank, mass density of water.
2. Note down the water level when pontoon is outside the tank.
3. Note down the water level when pontoon is inside the tank and their difference.
4. Fix the strips at equal distance from the center.
5. Put the weight on one of the hanger which gives the unbalanced mass.
6. Take the reading of the distance from center and angle made by pointer on arc.
7. The procedure can be repeated for other positioned and values of unbalanced mass.
Observations & Calculations:
Length of the tank =
Width of the tank =
Area of the tank =
Initial level of the water without pontoon =
Final level of the water without pontoon =
Difference in height of water(X) = X2 –X1 =
Height of water In tank with Pontoon (X2) (m) | Difference in Height X = X2-X1 (m) | Weight of Pontoon Wc = XAρ (kg) | Unbalanced Mass, Wm (kg) | Q | G M = Metacentric Height (m) | Xd (m) |
Conclusion: Meta centric height of the pontoon is measured with different positions and weights.
EXPERIMENT NO:10
Aim: To determine the minor losses due to sudden enlargement, sudden contraction and bend.
Apparatus: A flow circuit of G. I. pipes of different pipe fittings viz. Large bend, Small bend, Elbow, Sudden enlargement from 25 mm dia to 50 mm dia, Sudden contraction from 50 mm dia to 25 mm dia, U-tube differential manometer, collecting tank.
Theory:
Minor Losses: The local or minor head losses are caused by certain local features or disturbances .The disturbances may be caused in the size or shape of the pipe. This deformation affects the velocity distribution and may result in eddy formation.
Losses at bends, elbows and other fittings: The flow pattern regarding separation and eddying in region of separations in bends, valves. The resulting head loss due to energy dissipation can be prescribed by the relation h = KV2/2g. Where V is the average flow velocity and the resistance coefficient K depends on parameter defining the geometry of the section and flow.
Resistances of large sizes elbows can be reduced appreciably by splitting the flow into a number of streams by a jet of guide vanes called cascades.
Procedure:
1. Note down the relevant dimensions as diameter and length of pipe between the pressure tapping, area of collecting tank etc.
2. Pressure tapping of a pipe a is kept open while for other pipe is closed.
3. The flow rate was adjusted to its maximum value. By maintaining suitable amount of steady flow in the pipe.
4. The discharge flowing in the circuit is recorded together with the water level in the left and right limbs of manometer tube.
5. The flow rate is reduced in stages by means of flow control valve and the discharge & reading of manometer are recorded.
6. This procedure is repeated by closing the pressure tapping of this pipe, together with other pipes and for opening of another pipe.
Observations & Calculations:
Diameter of pipe D =
Length of pipe between pressure tapping L =
Area of collecting tank =
Types of the fitting =
| SNo | Manometer Reading | Discharge Measurement | Loss of coefficient K =(2g hL )/V2 | |||||
| Left limb h 1 (cm) | Right limb h 2 (cm) | Difference of head in terms of water hf =13.6(h1-h2) | Initial (cm) | Final (cm) | Time (sec) | Discharge Q (cm3/sec) | ||
| 1. | | | | | | | | |
| 2. | | | | | | | | |
| 3. | | | | | | | | |
| 4. | | | | | | | | |
Conclusion: Hence the minor losses due to sudden enlargement, sudden contraction and bend are determined.